P. B. Rathi* and V. K. Mourya1

1Department of Pharmaceutics, Shri Bhagwan College of Pharmacy, N-6, CIDCO, Aurangabad-431 003,
2Department of Pharmaceutics, Government College of Pharmacy, Vedant Hotel Road, Osmanpura, Aurangabad-431 005, India

Corresponding Author:
V. K. Mourya
Department of Pharmaceutics, Shri Bhagwan College of Pharmacy, N-6, CIDCO, Aurangabad-431 003
E-mail: pavanbrathi@gmail.com
Date of Submission 13 April 2010
Date of Revision 6 May 2011
Date of Acceptance 17 May 2011
Indian J. Pharm. Sci., 2011, 73 (3): 315-319  

DOI: 10.4103/0250-474X.93518

Abstract

The extended Hildebrand solubility parameter approach is used to estimate the solubility of satranidazole in binary solvent systems. The solubility of satranidazole in various dioxane-water mixtures was analyzed in terms of solutesolvent interactions using a modified version of Hildebrand-Scatchard treatment for regular solutions. The solubility of satranidazole in the binary solvent, dioxane-water shows a bell-shaped profile with a solubility maximum well above the ideal solubility of the drug. This is attributed to solvation of the drug with the dioxane-water mixture, and indicates that the solute-solvent interaction energy is larger than the geometric mean (δ1δ2) of regular solution theory. The new approach provides an accurate prediction of solubility once the interaction energy is obtained. In this case, the energy term is regressed against a polynomial in δ1 of the binary mixture. A quartic expression of W in terms of solvent solubility parameter was found for predicting the solubility of satranidazole in dioxane-water mixtures. The method has potential usefulness in preformulation and formulation studies during which solubility prediction is important for drug design.

Keywords

Dioxane, extended Hildebrand solubility approach, ideal solubility, interaction energy, regular solution theory, satranidazole, solubility parameter

Solubility data on drugs and pharmaceutical adjuncts in mixed solvents have wide applications in the drug sciences. Knowledge of interaction forces between solutes and solvents are of considerable theoretical and practical interest throughout the physical and biological sciences [1]. The theory of solution is one of the most challenging branch of physical chemistry. The Hildebrand-Scatchard theory of regular solution is the pioneer approach in this field, used to estimate solubility only for relatively non-polar drugs in nonpolar solvents [2]. An irregular solution is one in which self-association of solute or solvent, solvation of the solute by the solvent molecules, or complexation of two or more solute species are involved [3]. Polar systems exhibit irregular solution behaviour and are commonly encountered in pharmacy. The extended Hildebrand solubility approach (EHSA), a modification of the Hildebrand-Scatchard equation, permits calculation of the solubility of polar and non polar solutes in solvents ranging from non polar hydrocarbons to highly polar solvents such as water, ethanol, and glycols [4]. The solubility parameters of solute and solvent were introduced to explain the behaviour of regular and irregular solutions [5]. The extended Hildebrand solubility parameter approach has been developed to reproduce the solubility of drugs and other solids in the binary solvent systems [6].

The Hildebrand-Scatchard Eqn. for solubility of solids in a regular solution may be written as [7], 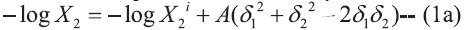and

The extended Hildebrand Eqn for the solubility of solids in an irregular solution may be written as [8]: 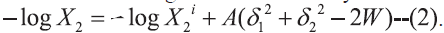From the geometric mean: 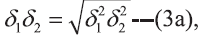where, in pharmaceutical solutions, the square root of geometric mean of δ12 and δ22, that is δ1δ2= (δ12δ22)1/2, is too restrictive and ordinarily provides a poor fit to experimental data in irregular solutions. The assumption that the geometric mean of two geometric parameters δ1δ2 (eqn 1a) can be replaced by a less restrictive term W (eqn 2), interaction energy parameter, which is allowed to take on values as required to yield correct mole fraction solubilities, X2 as [9], 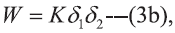Where, K is the proportionality factor relating interaction energy (W) to the geometric mean of solubility parameter (δ).

In Eqns 1 and 2, X2 and X2i are the mole fraction solubility and ideal mole fraction solubility of the solute respectively. The terms δ1 and δ2 are the solubility parameters for the solvent and solute respectively. The geometric mean, δ1δ2, provides a reasonable estimate of solvent-solute interaction in regular (ordinarily nonpolar) mixtures, whereas W or Kδ1δ2 is required to express solubility’s in nonregular systems (irregular solutions) of drugs in associating mixed solvents.

The term A in Eqns 1 and 2 is defined as [10]:

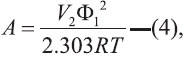
where, V2 is the molar volume of the solute taken as a supercooled liquid at solution temperature, R is the universal gas constant, T is the absolute temperature, 298.2 K, of the experiment and Φ1, the volume fraction of the solvent, is [11]: 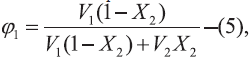where, V1 is the molar volume of the solvent at 25º.

Satranidazole, [1-methylsulphonyl-3-(1-methyl-5- nitro-2-imidazolyl)-2-imidazolidinone], a potent broad spectrum antiprotozoal, is very sparingly soluble in water (0.01mg/ml) [12,13]. It is not official in I.P., U.S.P. and B.P till date [14,15].

A perusal to the structure of satranidazole (fig. 1) indicates that the molecule is highly aromatic and the functional groups may not contribute much to the aqueous solubility. The poor aqueous solubility and wettability of satranidazole give rise to difficulties in pharmaceutical formulations meant for oral or parenteral use, which may lead to variation in bioavailability [16]. Therefore, it is necessary to explore the solubility of satranidazole in water-dioxane binary mixture.

Figure

Figure 1: Structure of satranidazole.

Dioxane is a very interesting cosolvent to study the interrelation between drug solubility and medium polarity because it is completely non-aqueous water miscible solvent [17]. Water-dioxane binary mixtures are strongly non ideal and can act in the solute-solvation process via hydrophobic interactions and preferential solvation because, water-dioxane mixtures cover a wide range of Hildebrand solubility parameters from 10.00 (Cal/cm3)0.5 (pure dioxane) to 23.4 (Cal/cm3)0.5 (pure water). Thus, satranidazole is an ideal candidate for the study of solubility behaviour in mixtures of dioxane and water. The present investigation pertains to the utility of EHSA in relation to the satranidazole solubility in the solvent pair dioxane-water binary solvents.

Satranidazole, obtained as gift sample from Alkem Laboratories Ltd., Baddi, India, was purified by recrystallization process. The solvent used for recrystallization of satranidazole was acetone. 1,4-Dioxane and acetone were obtained as gift sample from E. Merck, Ltd.; Mumbai, India and Qualigens Fine Chemicals, Mumbai, India, respectively. Throughout the study double distilled water was used for experimental purpose. All chemicals and reagents used in the study were of analytical grade and used as such. Double beam UV/Vis spectrophotometer, Shimadzu model 1601 with spectral bandwidth of 2 nm, wavelength accuracy ±0.5 nm and a pair of 10 mm matched quartz cells was used to measure absorbance of the resulting solutions. Citizen balance, CX-100, was used for weighing of Satranidazole.

The solubility of satranidazole was determined in binary solvent mixtures of dioxane and water. Double distilled water was used to prepare mixtures with dioxane in concentrations of 0-100% by volume of dioxane. About 10 ml of dioxane, water, or binary solvent was introduced into screw-capped vials containing an excess amount of satranidazole. After being sealed with several turns of electrical tape, the vials were submerged in water at 25±0.4° and were shaken at 150 rpm for 24 h in a constant-temperature bath. Preliminary studies showed that this time period was sufficient to ensure saturation at 25°.

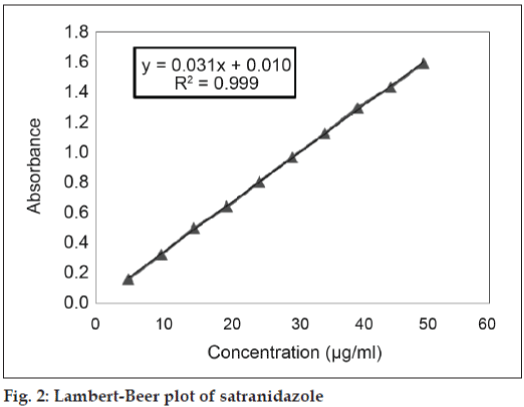
After equilibrium was attained, vials were removed for analysis. Firstly, solutions were filtered through Whatman filter paper (No. 41). After appropriate dilutions with double distilled water, the solutions were analyzed by using a spectrophotometer set at the wavelength of maximum absorption of the satranidazole (λmax-319.80 nm). Calibration graph of satranidazole in solvent blend was previously established with very high degree of correlation coefficient (R2) 0.9997, slope 0.0318 and negligible intercept (0.0101) as shown in fig. 2. The working concentration range was from 5 to 50 μg/ml. The solubility of the satranidazole was determined at least three times for each solvent, and the average value was taken. The densities of the solvent mixtures and the filtrates of the saturated solutions of satranidazole were determined in triplicate at 25±0.4° using 10-ml specific gravity bottle.

Figure

Figure 2: Lambert-Beer plot of satranidazole.

The solubility parameters of the solvents were obtained from the literature [18]. The solubility parameter of satranidazole was calculated by the method of Fedor [19,20], which was confirmed by solubility analysis in dioxane-water blend.

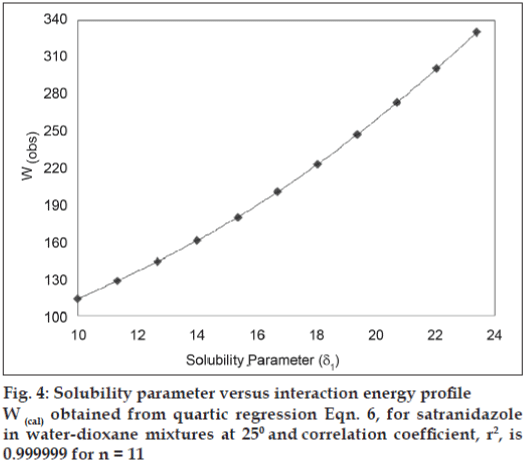
Experimental data of mole fraction solubility of satranidazole in dioxane-water are plotted against solubility parameters of solvent blend (fig. 3) exhibit a maxima at δ1= 11.34 (peak solubility = 0.0096347 mol/l). The observed solubility is comparatively higher than the ideal solubility (X2i= 0.00245614 mol/l). According to the regular solution theory, solubility cannot exceed ideal solubility. However, in non regular solutions, peak solubility may depart from ideal solubility due to solute solvent interactions. This abnormal behavior has been dealt with the theoretical replacement of mean geometric solubility parameters (δ1δ2) term with the interaction energy term (W). To relate these two variables, a fourth power polynomial (quartic expression) has been developed to back calculate the value of Wcal. For Dioxane-water system, the polynomial has following values: Wcal= 21.70104+10.372731 δ1-0.401841 δ12 +0.032507δ13 -0.000435δ14, (n=11, R2= 0.9999) -- (6)

Figure

Figure 3: Solubility of satranidazole in dioxane, water, and dioxanewater mixtures Solubility of satranidazole in dioxane, water, and dioxane-water mixtures at 250. Key: ♦ Experimental solubilities and back-calculated solubilities from Eq. 2.

These polynomials are used successfully for the calculation of W, at any value of solubility parameter (δ1), which was subsequently employed to calculate mole fraction solubility of solute in a solvent blend using backward regression. Representative data along with validation parameters are summarized in Table 1. Wcal values are indicating significant interaction of satranidazole and solvent molecules at the peak of solubility profile.

Water: Dioxane(%v/v) Solubility(g/ml) δ1(Cal/cm3)0.5 V1 Density of blend Mol. Wt of blend X2(obs) W(obs)
100:0 0.0005821 23.40 18.00 0.9980 18.00 3.6317E-05 330.45
90:10 0.0009112 22.06 24.77 1.0014 25.01 7.8740E-05 300.96
80:20 0.0012872 20.72 31.54 1.0048 32.02 1.4198E-04 273.03
70:30 0.0020104 19.38 38.31 1.0082 39.03 2.6954E-04 246.97
60:40 0.0039050 18.04 45.08 1.0116 46.04 6.1647E-04 222.93
50:50 0.0064722 16.70 51.85 1.0150 53.06 1.1757E-03 200.45
40:60 0.0109196 15.36 58.62 1.0184 60.07 2.2456E-03 179.77
30:70 0.0180788 14.02 65.39 1.0218 67.08 4.1594E-03 160.85
20:80 0.0286006 12.68 72.16 1.0252 74.09 7.2968E-03 143.66
10:90 0.0344943 11.34 78.93 1.0286 81.10 9.6347E-03 127.92
0:100 0.0262503 10.00 85.70 1.0320 88.11 7.8876E-03 113.38

Table 1: Molar observed solubility and calculation parameters of satranidazole in dioxanewater mixtures

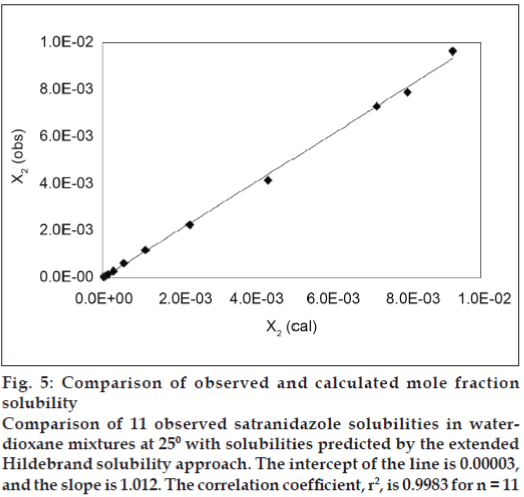
Observed solubility data was subjected to the evaluation of interaction energy. Experimental values of interaction energy (Wobs) were regressed against solubility parameter to obtain Wcal (fig. 4), which was then used to back calculate the mole fraction solubility (X2cal). A mathematical model is proposed for individual system as fourth power polynomial. Validation of this equation has been done by comparing experimentally obtained and calculated values of mole fraction solubility by estimating residuals and percent difference (Table 2). The Extended Hildebrand Approach applied to the solubility data of satranidazole in waterdioxane mixtures, which reproduces the satranidazole solubility within the accuracy ordinarily achieved in such measurements. The predictive capability of the model for satranidazole is represented in fig.5, which indicates a very high degree of correlation coefficient (R2) 0.9983 and negligible intercept (-0.00003) equal to zero.

Figure

Figure 4: Solubility parameter versus interaction energy profile W (cal) obtained from quartic regression Eqn. 6, for satranidazole in water-dioxane mixtures at 250 and correlation coefficient, r2, is 0.999999 for n = 11

Figure

Figure 5: Comparison of observed and calculated mole fraction solubility Comparison of 11 observed satranidazole solubilities in waterdioxane mixtures at 250 with solubilities predicted by the extended Hildebrand solubility approach. The intercept of the line is 0.00003, and the slope is 1.012. The correlation coefficient, r2, is 0.9983 for n = 11

W(obs) W(cal) X2(obs) X2(cal) logγ2/A(obs) logγ2/A(cal) Residual Percentdifference
330.447760 330.457908 3.6317E-05 3.6612E-05 16.396580 16.376285 -8.0988E-03 -8.10E-01
300.959157 300.911658 7.8740E-05 7.5824E-05 14.457387 14.552385 3.7032E-02 3.70E+00
273.034580 273.083225 1.4198E-04 1.4757E-04 12.981340 12.884050 -3.9377E-02 -3.94E+00
246.968191 247.040724 2.6954E-04 2.8550E-04 11.380118 11.235053 -5.9205E-02 -5.92E+00
222.925499 222.818600 6.1647E-04 5.6651E-04 9.322702 9.536501 8.1035E-02 8.10E+00
200.450067 200.417633 1.1757E-03 1.1460E-03 7.721966 7.786834 2.5208E-02 2.52E+00
179.769542 179.804938 2.2456E-03 2.3086E-03 6.122616 6.051824 -2.8046E-02 -2.80E+00
160.846625 160.913961 4.1594E-03 4.3814E-03 4.599250 4.464578 -5.3374E-02 -5.34E+00
143.657648 143.644482 7.2968E-03 7.2243E-03 3.199204 3.225536 9.9401E-03 9.94E-01
127.918366 127.862615 9.6347E-03 9.2394E-03 2.490968 2.602470 4.1024E-02 4.10E+00
113.375305 113.400807 7.8876E-03 8.0423E-03 2.981490 2.930486 -1.9622E-02 -1.96E+00

Table 2: Comparisons of observed and calculated mole fraction solubilities of satranidazole in dioxane-water mixtures at 25°

On the basis of validation parameters, it can be expressed that the behavior of non regular solution can be quantified more precisely using EHSA. The procedure can be explored further to predict the solubility of satranidazole in pure water or dioxane and in any water-dioxane mixtures. Simultaneously, this tool may become useful in optimization problems of clear solution formulations. Thus the method has potential usefulness in preformulation and formulation studies during which solubility prediction is important for drug design.

Acknowledgements

Authors wish to express their gratitude to M/S Alkem Laboratories Limited, Baddi for providing gift sample of Satranidazole. Authors are also thankful to E. Merck, Ltd.; Mumbai, India and Qualigens Fine Chemicals, Mumbai, India for providing gift sample of 1,4-Dioxane and Acetone respectively.

References